Estimating Taxon-Environment Relationships: Placing Taxa into Tolerance Categories
Placing Taxa into Tolerance Categories
Taxa can be classified into three groups on the basis of the shape of their taxon-environment relationship: (1) monotonically increasing, (2) monotonically decreasing, or (3) unimodal (Yuan 2004). Then, when an increasing value of the environmental variable can be attributed to human activities, those taxa with monotonically increasing taxon-environment relationships are designated as tolerant, and those with monotonically decreasing relationships are designated as sensitive. Taxa with unimodal taxon-environment relationships are designated as intermediately tolerant.
A graphical approach to curve classification is required for nonparametric regressions. The maximum modeled occurrence probability, in this case, is compared with the confidence limits on either side of the taxon optima. If a horizontal line drawn through the maximum mean occurrence probability deviates from the upper confidence limits on both sides of the taxon optima (e.g., Malenka in Figure 15), then the taxon is designated as unimodal. If the line deviates from the upper confidence limit only on the right-hand side of the taxon optimum (e.g., Heterlimnius in Figure 15), then the taxon is designated as a decreaser. If it deviates only on the left-hand side, then the taxon is designated as an increaser. A parallel set of conditions can be specified for the lower confidence limit. Assuming that human activities cause an increase in the value of the environmental gradient, increasers would be identified as tolerant taxa, decreasers would be identified as intolerant taxa, and unimodal taxa would be designated as intermediately tolerant.
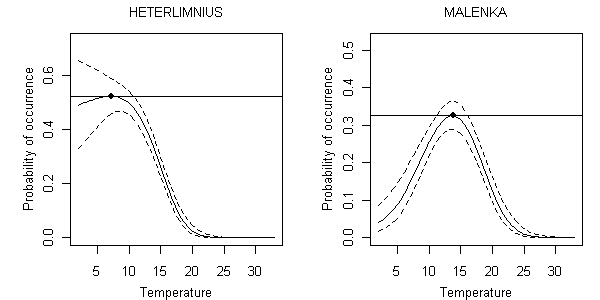 Figure 15. Graphical approach for classifying curve shape for Heterlimnius and Malenka. Solid line is mean response, dotted lines are estimated 90% confidence limits about mean response, solid horizontal line is location of maximum mean response for comparison with confidence limits. Horizontal axis in units of degrees C.
Figure 15. Graphical approach for classifying curve shape for Heterlimnius and Malenka. Solid line is mean response, dotted lines are estimated 90% confidence limits about mean response, solid horizontal line is location of maximum mean response for comparison with confidence limits. Horizontal axis in units of degrees C.
Curve classifications allow taxa to be broadly characterized as tolerant or sensitive to changes in the value of specific environmental gradients. These tolerance classifications can then be useful for computing biological metrics. Statistical scripts for defining curve shape are available under the R Scripts tab of this Appendix.
References
- U.S. EPA (2006) Estimation and Application of Macroinvertebrate Tolerance Values (PDF) (89 pp, 1.69 Mb, About PDF) (EPA/600/P-04/116F) Office of Research and Development, Washington, DC.
- Yuan LL (2004) Assigning macroinvertebrate tolerance classifications using generalised additive models. Freshwater Biology 49:662-677.
